Alt text:
So what do we do when we get to base 10? Do we use A, B, C, etc? No: Numbers larger than about 3.6 million are simply illegal.
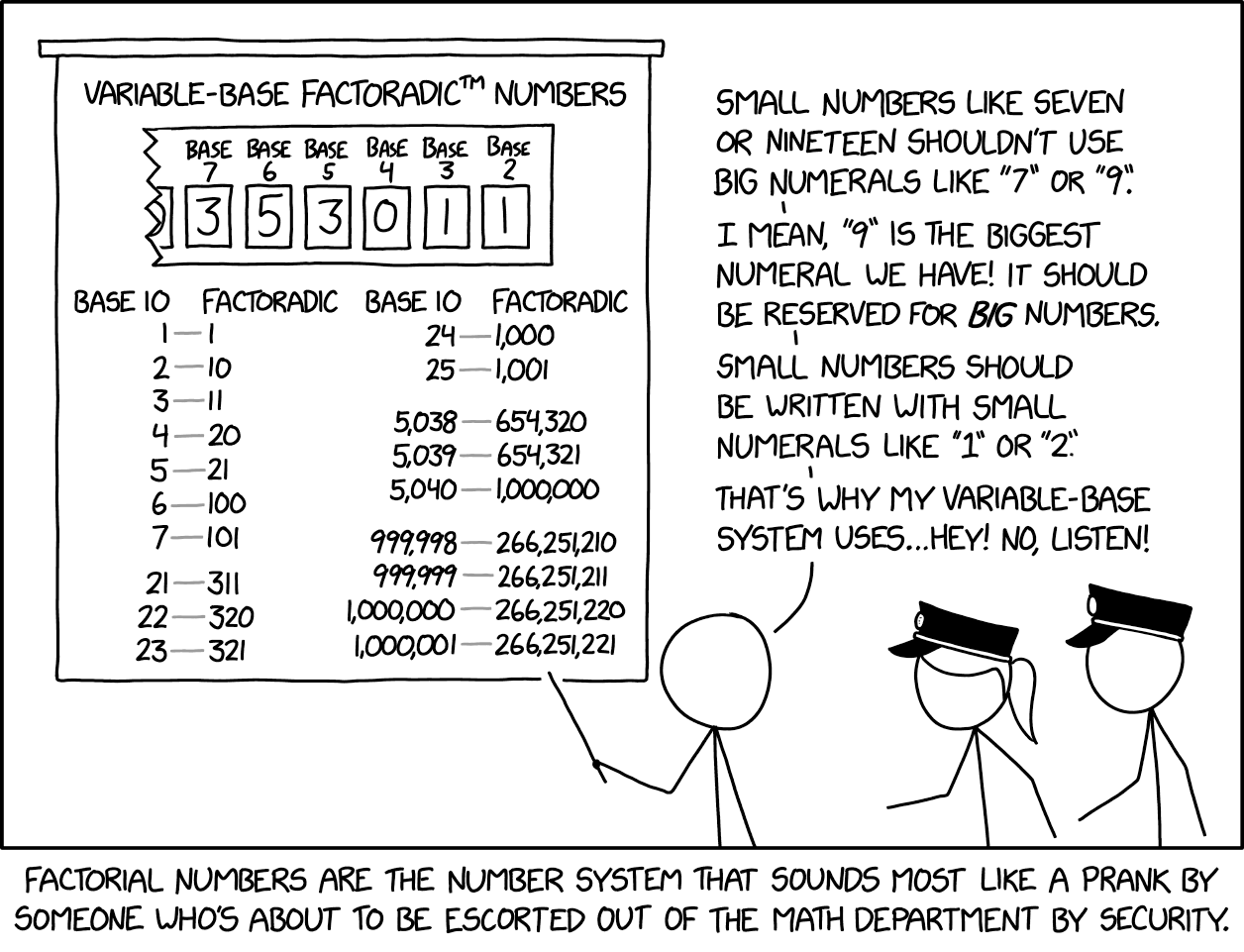
Finally, a system that uses more information to express less information.
According to this article, the factoradical system gets efficient for numbers larger than 20!, but i guess this here is a shining example of ~~less is~~ more is less
It begins to improve related to regular base-10 after, well, 10!, but it takes a while to recover for lower base numbers before that.
Good grief, it's far too early in the morning for this sort of thing. My brain hurts now.
This is cursed, haha
What's the point of such a system ?
Hum... Have you checked what site it's on?
Idk trolling
yes
0 = 0
1 = 1
2 = 10
3 = 11
4 = 20
5 = 21
6 = 100
101, 110, 111, 120, 121,
200, 201, 210, 211, 220, 221, 300, 301...
Amidoinitrite
This is actually a pretty cool idea.
Not really. The reality is that the only real metric for the utility of a notation is the speed of computation. A constant positional notation system is the most efficient, then you just optimise for a base whose multiplication table can be memorised (27 is a good one). Many people are under the impression that highly composite bases are better, but the reality is that it only optimises for euclidean division which is far out weighed by multiplication and addition (and can be easily computed using them).
A community for a webcomic of romance, sarcasm, math, and language.